High-Order Accurate Solutions of the Point Kinetics Equations with the Spectral Deferred Correction Method

Solving initial value problems with high-order methods receives considerable attention in many fields because these methods can potentially improve the accuracy of the simulation results with lower computational cost than low-order methods. Most methods, however, are either complicated to implement or unstable when the order of accuracy is high. The spectral deferred correction (SDC) method is a stable, robust, and efficient high-order time-integration scheme capable of an arbitrary order of accuracy. In this paper, we apply the SDC method to solve the initial value problem of the point kinetics equations (PKEs). For our implementation, we show that SDC is 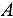-stable for orders up to eight and the order of accuracy is verified for PKE problems with a range of different reactivities. A fifth-order SDC method was then implemented to solve the exact PKE in the transient multilevel method of MPACT. The error from solutions of the exact PKE with SDC is shown to be negligible. The investigations made here can provide the foundation for future investigations simulating the neutron transport problem using the high-order methods for both spatial discretization and time integration.