Benchmark Solutions for Radiative Transfer with a Moving Mesh and Exact Uncollided Source Treatments

The set of benchmark solutions used in the thermal radiative transfer community suffers some coverage gaps, in particular, nonlinear, nonequilibrium problems. Also, there are no nonequilibrium, optically thick benchmarks. These shortcomings motivated the development of a numerical method free from the requirement of linearity and easily able to converge on smooth optically thick problems, i.e., a moving mesh Discontinuous Galerkin framework that utilizes an uncollided source treatment. Having already proven this method on time-dependent scattering transport problems, we present here solutions to nonequilibrium thermal radiative transfer problems for familiar linearized systems together with more physical nonlinear systems in both optically thin and thick regimes, including both the full transport and the 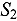/ 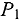solution. Geometric convergence is observed for smooth sources at all times and some nonsmooth sources at late times when there is local equilibrium. Also, accurate solutions are achieved for step sources when the solution is not smooth.