Variable Dynamic Mode Decomposition for Estimating Time Eigenvalues in Nuclear Systems

We present a new approach to calculating time eigenvalues of the neutron transport operator (also known as 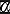eigenvalues) by extending the dynamic mode decomposition (DMD) to allow for nonuniform time steps. The new method, called variable dynamic mode decomposition (VDMD), is shown to be accurate when computing eigenvalues for systems that were infeasible with DMD due to a large separation in timescales (such as those that occur in delayed supercritical systems). The 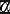eigenvalues of an infinite medium neutron transport problem with delayed neutrons, and consequently having multiple, very different relevant timescales, are computed. Furthermore, VDMD is shown to be of similar accuracy to the original DMD approach when computing eigenvalues in other systems where the previously studied DMD approach can be used.