On the Regularity Order of the Pointwise Uncollided Angular Flux and Asymptotic Convergence of the Discrete Ordinates Approximation of the Scalar Flux

To determine the angular discretization error asymptotic convergence rate of the uncollided scalar flux computed with the discrete ordinates (S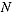) method, a comprehensive theory of the regularity order with respect to the azimuthal angle of the exact pointwise S_N uncollided angular flux is derived based on the integral form of the transport equation in two-dimensional Cartesian geometry. With this theory, the regularity order of the pointwise 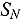uncollided angular flux can be estimated for a given problem configuration. Our new theory inspired a novel Modified Simpson’s (MS) quadrature that converges the uncollided scalar flux faster than any of the traditional quadratures by avoiding integration across points of irregularity in the azimuthal angle. Numerical results successfully verify our new theory in four variants of a test configuration, and the angular discretization errors in the corresponding scalar flux computed with conventional angular quadrature types and with our new quadrature types are found to converge with different orders. The error convergence rates obtained with traditional quadrature types are limited by the regularity order of the exact angular flux and the quadrature’s integration intervals while our new MS quadrature types converge with order two to four times higher than traditional quadratures. A detailed study of oscillations observed in certain quadrature errors is provided by introducing the effective length of the irregular interval and the associated oscillating function.