The Time-Dependent Asymptotic P_N Approximation for the Transport Equation

In this study, a spatio-temporal approach for the solution of the time-dependent Boltzmann (transport) equation is derived. Finding the exact solution using the Boltzmann equation for the general case is generally an open problem and approximate methods are usually used. One of the most common methods is the spherical harmonics method (the 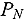approximation), when the exact transport equation is replaced with a closed set of equations for the moments of the density with some closure assumption. Unfortunately, the classic 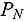closure yields poor results with low-order N in highly anisotropic problems. Specifically, the tails of the particles’ positional distribution as attained by the approximation are inaccurate compared to the true behavior. In this work, we present a derivation of a linear closure that even for low-order approximation yields a solution that is superior to the classical 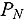approximation. This closure is based on an asymptotic derivation both for space and time of the exact Boltzmann equation in infinite homogeneous media. We test this approximation with respect to the one-dimensional benchmark of the full Green function in infinite media. The convergence of the proposed approximation is also faster when compared to (classic or modified) 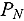approximation.