Monte Carlo Perturbation Theory Estimates of Sensitivities to System Dimensions

Monte Carlo methods are developed using adjoint-based perturbation theory and the differential operator method to compute the sensitivities of the k-eigenvalue, linear functions of the flux (reaction rates), and bilinear functions of the forward and adjoint flux (kinetics parameters) to system dimensions for uniform expansions or contractions. The calculation of sensitivities to system dimensions requires computing scattering and fission sources at material interfaces using collisions occurring at the interface—which is a set of events with infinitesimal probability. Kernel density estimators are used to estimate the source at interfaces using collisions occurring near the interface. The methods for computing sensitivities of linear and bilinear ratios are derived using the differential operator method and adjoint-based perturbation theory and are shown to be equivalent to methods previously developed using a collision history–based approach. The methods for determining sensitivities to system dimensions are tested on a series of fast, intermediate, and thermal critical benchmarks as well as a pressurized water reactor benchmark problem with iterated fission probability used for adjoint-weighting. The estimators are shown to agree within 5% and 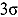of reference solutions obtained using direct perturbations with central differences for the majority of test problems.