Dead-Time Effects on Counting Statistics in Subcritical Nuclear Systems

The analysis of the fluctuations of signals coming from detectors in the vicinity of a subcritical assembly of fissile materials is commonly used for the control and safeguard of nuclear materials and might be used for the surveillance of an accelerator driven system. One of the stochastic techniques is the measurement of the probability distributions of counts in time intervals 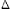t (gates); the departure of the ratio of the variance and the mean value with respect to 1 (the correlation) is directly related to the amount of fissile material and its subcriticality. The measurement of this correlation is affected by dead-time effects due to count losses because of the finite-time resolution of the detection system. We present a theory that allows (a) the calculation of the probability of losing n counts (P⁽ⁿ⁾) in gate 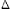t, (b) the definition of experimental conditions under which P⁽²⁾ << P⁽¹⁾, and (c) a methodology to correct the measured correlation because of losing one count in any gate. The theory is applied to the analysis of experiments performed in a highly enriched subcritical assembly.